Probability is a way of expressing knowledge or belief that an event will happen or has already happened. The concept gained exact mathematical significance in a theory that is widely used in research areas such as mathematics, statistics, finance, gambling, science and philosophy to draw conclusions about the possibility of potential events and the underlying mechanics of complex systems. The word "probability" does not have an agreed direct definition. In fact, there are two broad categories of interpretations, adherents of which have different views on its fundamental nature. In this article you will find a lot of useful information for yourself, discover mathematical concepts, find out what probability is measured in and what it represents.
Types of probability
What is measured?
There are four types, each with its own limitations. None of these approaches are wrong, but some are more useful or more general than others.
- Classical probability. This interpretation owes its name to the early and August genealogy. Defended by Laplace and found even in the works of Pascal, Bernoulli, Huygens and Leibniz, it assigns probability in the absence of any or in the presence of symmetrically balanced evidence. The classical theory is applicable to equiprobable events, such as the outcome of a coin or dice roll. Such events were known as equipossible. Probability = number of favorable equipossibilies / total number of corresponding equipossibilities.
- Logical probability. Logical theories preserve the idea of a classical interpretation that they can be determined a priori by exploring the space of possibilities.
Subjective probability. Which is obtained from a person’s personal judgment about whether a specific result can occur. It does not contain formal calculations and reflects only opinions
Some of the examples of probability
In what units is the probability measured:
- X says, "Don't buy avocados here. About half the time they are rotten." X expresses his belief about the likelihood of the event - that the avocado will be rotten - based on his personal experience.
- Y says: "I’m 95% sure that the capital of Spain is Barcelona." Here faith Y expresses probability from his point of view, because only he does not know that Madrid is the capital of Spain (in our opinion, the probability is 100%). However, we can consider it as subjective, since it expresses a measure of uncertainty. It is as if Y said: "In 95% of cases, when I feel as confident as in this, I am right."
- Z says: "The probability of being shot in Omaha is lower than in Detroit." Z expresses a belief based (presumably) on statistics.
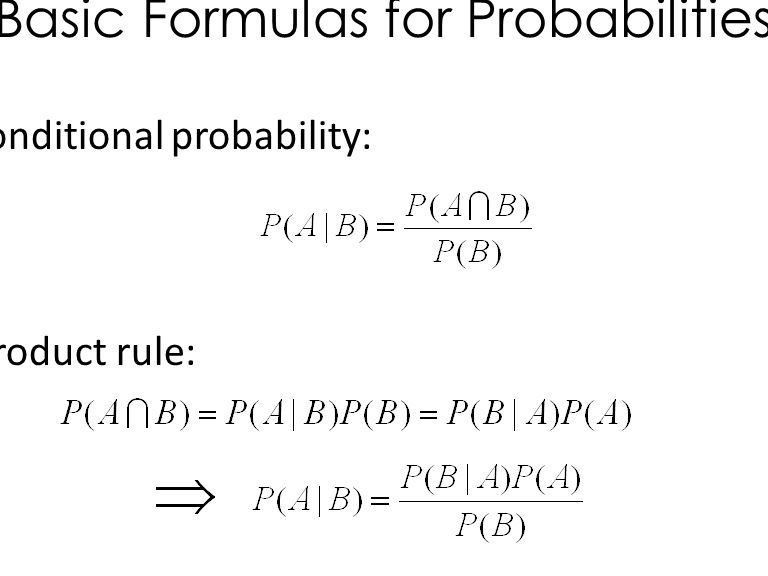
Mathematical processing
What is probability measured in math?
In mathematics, the probability of an event A is represented by a real number in the range from 0 to 1 and is written as P (A), p (A) or Pr (A). An impossible event has a chance of 0, and a definite one has 1. However, this is not always true: the probability 0 of an event is impossible, just as 1. The opposite or complement of event A is event not A (that is, event A that does not occur). Its probability is determined by P (not A) = 1 - P (A). As an example, the possibility of not rolling six on a hexagonal matrix is 1 - (chance of rolling six). If both events A and B occur on the same experiment, this is called the intersection, or the joint probability of A and B. For example, if two coins flip over, there is a chance that both will fall out. If event A, or B, or both occur on the same run of an experiment, this is called combining events A and B. If two events are mutually exclusive, then the probability of their occurrence is equal.
I hope that now we have answered the question of what is the probability measured.
Conclusion
The revolutionary discovery of 20th-century physics was the random nature of all physical processes occurring on a subatomic scale and obeying the laws of quantum mechanics. The wave function itself develops deterministically until no observations are made. But, according to the prevailing Copenhagen interpretation, randomness caused by the collapse of the wave function during observation is fundamental. This means that probability theory is necessary to describe nature. Others have not come to terms with the loss of determinism. Albert Einstein famously remarked in a letter to Max Bourne: "I am convinced that God does not play dice." Although there are alternative points of view, such as quantum decoherence, which is the cause of seemingly random collapse. There is now a strong consensus among physicists that probability theory is necessary to describe quantum phenomena.